Understanding the Implied Volatility Surface
- Aki Kakko

- Mar 16, 2024
- 4 min read
The implied volatility surface is a crucial concept for investors who trade options or need to properly account for options positions. It provides a visualization of the implied volatility of options across different strike prices and expiration dates for a given underlying asset. Implied volatility represents the market's expectation of how volatile the price of the underlying asset will be until the option expires. It is one of the key inputs used in option pricing models like the Black-Scholes model, along with other factors like the underlying price, strike price, time to expiration, and risk-free rate. The implied volatility surface plots implied volatility on the vertical axis against the strike price on the horizontal axis, with different expiration dates shown as individual curved lines. This allows investors to analyze the volatility smile or skew across different strikes and expirations.
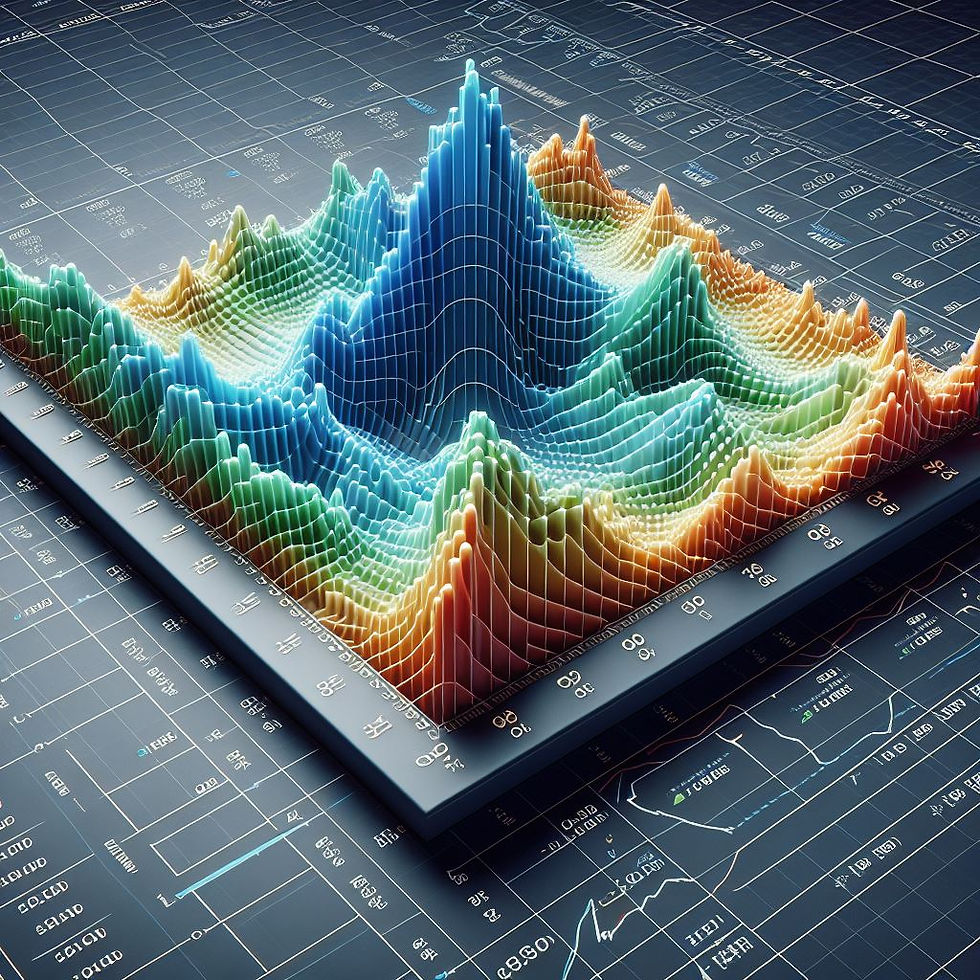
For many underlying assets, the implied volatility surface exhibits a volatility smile or skew pattern. A volatility smile means that options with strike prices furthest away from the current underlying price (both calls and puts) have higher implied volatilities. For example, let's say XYZ stock is trading at $100. The implied volatility surface may show that XYZ options with strikes of $80 and $120 have higher implied volatilities than at-the-money $100 strikes. This smile pattern suggests the market expects a greater probability of extreme moves in the underlying price. Conversely, a volatility skew means that either call or put options have systematically higher implied volatilities compared to the other side. This often happens for equity indexes and individual stocks, where put options tend to have higher implied volatilities due to crash risk fears.
Reading the Volatility Surface
By analyzing the shape, level, and dynamics of the implied volatility surface, investors can gain insights into:
Overall market sentiment and expectations around future volatility
Relative pricing of options across strikes/expirations
Supply and demand dynamics in the options market
For example, a steeply sloped volatility surface indicates that the market expects the underlying price to be range-bound. Monitoring changes in volatility surfaces can also alert investors to potential dislocations or mispricing of options.
Term Structure Effects
The term structure refers to how implied volatilities change with time to expiration. Typically, longer-dated options have higher implied volatilities due to greater uncertainty over longer time frames. However, this can vary based on market conditions. A downward-sloping term structure (higher short-dated IV) may indicate investors expect an imminent volatility spike and are demanding more premium for near-term protection. Conversely, an upward term structure suggests greater uncertainty in the long run.
Factors Driving the Surface
The implied volatility surface is driven by a combination of factors like:
Expected future volatility in the underlying price
Supply and demand for options at different strikes
Investor sentiment and risk preferences
Hedging flows from institutions
Replication of volatility exposures by market makers
Event risk, earnings announcements, and other catalysts can also dramatically impact volatility surfaces leading into and following such events.
Modeling the Surface
While the classic Black-Scholes model assumes constant implied volatility across all strikes and expirations, more sophisticated option pricing models attempt to directly model the implied volatility surface itself. Popular models include:
Stochastic Volatility Models: These allow the volatility parameter to evolve stochastically over time based on its own process, producing a richer volatility surface.
Local Volatility Models: These allow volatility to vary based on both the current asset price and time to expiration.
Practitioner Black-Scholes: This model applies moneyness and time adjustments to the Black-Scholes formula to better match observed volatility skew/smile.
Using appropriate volatility surface models is important for accurate pricing, hedge ratios, and risk management of options portfolios.
Surface Dynamics
How the volatility surface shifts and evolves over time provides signals about changing market conditions:
Volatile Environments: When markets are volatile, the entire volatility surface tends to become elevated and exhibit a more pronounced smile, reflecting higher probabilities of large moves priced in.
Market Crashes: Following a major sell-off, volatility surfaces often develop a sharp negative skew, with puts becoming extremely expensive as investors brace for further downside.
Calm Markets: Extended periods of low volatility can cause volatility surfaces to flatten as options become cheaper to reflect lower expected swings.
Monitoring surface dynamics proactively helps anticipate potential volatility regime shifts.
Relative Value Strategies
Some investors look to identify rich/cheap areas of the volatility surface to execute relative value trades. For example:
Selling overpriced short-dated put options to exploit a steeply negative front volatility skew.
Putting on risk reversals that sell expensive out-of-the-money puts and buy cheap out-of-the-money calls to position for a volatility event.
Delta-hedging these positions aims to isolate and profit from implied volatility divergences while remaining market-neutral.
The volatility surface factors heavily into the pricing and risk management of such volatility arbitrage strategies used by hedge funds and market makers. While complex, developing an intuitive understanding of the implied volatility surface is invaluable for any investor looking to effectively navigate options markets across different market conditions. Its multi-dimensional insights on volatility can drive more informed trading and risk management decisions.
Properly understanding and interpreting the implied volatility surface is critical for investors looking to optimize option trading strategies, manage volatility risk exposures, or identify potential mispriced options. The surface provides a wealth of information about market expectations and relative pricing across strike prices and expirations.



Comments